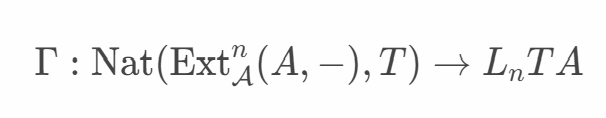Homological Yoneda Lemma

Yoneda lemma的同调代数版本. 这是个很神奇的事情, 可以通过natural transformation 和Ext函子来计算一些函子的左导出函子. 整体的证明参考了论文The Yoneda isomorphism commutes with homology

Homological Yoneda Lemma

In $\mathrm{Mod}$ category. Let $0\to R_{n-1}\to P_{n-1}\to\cdots\to P_{0}\to A\to 0$ be an exact sequence with $P_{n-1},\dots,P_{0}$ projective. Let $T$ be an addictive functor. Define $\tilde{L}_{n}TA=\mathrm{Ker}(TR_{n-1}\to TP_{n-1})$ . Then we have $\tilde{L}_{n}TA=L_{n}TA$ , the left derived functor of $T$ .

Theorem 1. Let $T: \mathrm{Mod}\to \mathcal{A}$ be an addictive covariant functor, $T$ right exact and $A$ be an module. Then there are natural isomorphism

\Gamma:\mathrm{Nat}(\mathrm{Ext}_{\mathcal{A}}^{n}(A,-),T)\to L_{n}TA

Proof

Proof. We prove the functor $H^{n}$ commutes with Yoneda embedding, i.e. $\mathrm{Nat}(H^{n}\mathrm{Hom}(A,-),T)\xrightarrow{\cong}H_{n}TA$ . Here $A$ is a chain complex

\cdots\to A_{n+1}\xrightarrow{d_{n+1}}A_{n}\xrightarrow{d_{n}}A_{n-1}\xrightarrow{d_{n-1}}\cdots

and

\cdots\to \mathrm{Hom}(A_{n-1},-)\xrightarrow{d_{n}^{*}}\mathrm{Hom}(A_{n})\xrightarrow{d_{n+1}^{*}}\mathrm{Hom}(A_{n+1})\to \cdots

We compute $\mathrm{Nat}(H^{n}\mathrm{Hom}(A,-),T)$ .

\begin{aligned} \mathrm{Nat}(H^{n}\mathrm{Hom}(A,-),T) &\cong \mathrm{Nat}(\mathrm{Coker}(\mathrm{Hom}(A_{n-1},-)\to \mathrm{Ker}(d_{n+1}^{*})),T)\\&\cong \mathrm{Ker}(\mathrm{Nat}(\mathrm{Ker}(d_{n+1}^{*}),T)\to \mathrm{Nat}(\mathrm{Hom}(A_{n-1},-),T))\\&\cong \mathrm{Ker}(\mathrm{Nat}(\mathrm{Hom}(\mathrm{Coker}(d_{n+1}),-),T)\to \mathrm{Nat}(\mathrm{Hom}(A_{n-1},-),T))\\& \cong \mathrm{Ker}(T\mathrm{Coker}(d_{n+1}\to TA_{n-1}))\\& \cong \mathrm{Ker}(\mathrm{Coker}(Td_{n+1})\to TA_{n-1})=H_{n}TA\end{aligned}

The last to isomorphism is given by Yoneda lemma and right exactness, respectively. If we do not have $T$ right exact, then we have $\mathrm{Ker}(T\mathrm{Coker}(d_{n+1})\to TA_{n-1})\cong \tilde{L}_{n}TA$ . ◻

Proposition 1. Every natural transformation $\phi:\mathrm{Ext}_{\mathcal{A}}^{1}(A,-)\to \mathrm{Ext}_{\mathcal{A}}^{1}(A',-)$ is induced by a morphism $f:A'\to A$ .

Proof

Proof. We have $\mathrm{Nat}(\mathrm{Ext}_{\mathcal{A}}^{1}(A,-)\to \mathrm{Ext}_{\mathcal{A}}^{1}(A',-))\cong \tilde{L}_{1}(\mathrm{Ext}_{\mathcal{A}}^{1}(A',-))(A)$ . For $0\to R\to P\to A\to 0$ a projective presentation of $A$ ,

\tilde{L}_{1}(\mathrm{Ext}_{\mathcal{A}}^{1}(A',-))(A)=\mathrm{Ker}(\mathrm{Ext}_{\mathcal{A}}^{1}(A',R)\to\mathrm{Ext}_{\mathcal{A}}^{1}(A',P))

From the long exact sequence theorem, we have

\cdots\to \mathrm{Hom}(A',P)\to \mathrm{Hom}(A',A)\to \mathrm{Ext}_{\mathcal{A}}^{1}(A',R)\to \mathrm{Ext}_{\mathcal{A}}^{1}(A',P)\to \cdots

Thus any natural transformation $\theta$ is mapped by an element $f$ in $\mathrm{Hom}(A',A)$ .

We omit the proof of the proposition: $\theta$ is indeed induced by $f$ . See Hilton & Stammbach. ◻